Vedic Mathematical Formulae
(वैदिक गणित के सूत्र)
Sutras
1. Ekadhikena Purvena (एकाधिकेन पूर्वेण)
पहले से एक अधिक के द्वारा।
‘By one more than the previous one’
Corollary: Anurupyena
2. Nikhilam navatascaramam Dasatah (निखिलम् नवतश्चरमं दशतः)
सभी नौ में से परन्तु अन्तिम दस में से।
‘All from nine and last from ten’
Corollary: Sisyate Sesasamjnah
3. Urdhva – tiryagbhyam (ऊर्ध्व तिर्यग्भ्याम्)
सीधे और तिरछे दोनों प्रकार से।
‘Vertically and crosswise’
Corollary: Adyamadyenantyamantyena
4. Paravartya Yojayet (परावर्त्य योजयेत्)
पक्षान्तरण कर उपयोग में लेना।
‘Transpose and apply’
Corollary: Kevalaih Saptakam Gunyat
5. Sunyam SamyaSamuccaye (शून्यं साम्य समुच्चये)
समुच्चय समान होने पर शून्य होता हैं।
‘When the samuchayas are same, then it is Zero’
Corollary: Vestanam
6. Anurupye – Shunyamanyat (आनुरूप्ये शून्यमन्यत्)
अनुरूपता होने पर दूसरा शून्य होता हैं।
‘If one is in ratio, the other one is zero’
Corollary: Vestanam
7. Sankalana – Vyavakalanabhyam (संकलन-व्यवकलनाभ्याम्)
जोड़कर और घटाकर।
‘By addition and subtraction’
Corollary: Yavadunam Tavadunikritya Vargancha Yojayet
8. Puranapuranabhyam (पूरणापूरणाभ्याम्)
अपूर्ण को पूर्ण करके।
‘By completing’
Corollary: Antyayordashake'pi
9. Chalana - Kalanabhyam (चलन-कलनाभ्याम्)
चलन-कलन के द्वारा।
‘By calculus’
Corollary: Antyayoreva
10. Yavadunam (यावदूनम्)
जितना कम हो, अर्थात् विचलन।
‘By the deficiency’
Corollary: Samuccayagunitah
11. Vyastisamastih (व्यष्टिसमष्टिः)
एक को पूर्ण तथा पूर्ण को एक मानते हुए।
‘Whole as one and one as whole’
Corollary: Lopanasthapanabhyam
12. Sesanyankena charamena (शेषाण्यड्केन चरमेण)
अंतिम अंक से अवशेष को।
‘Reminder by the last digit’
Corollary: Vilokanam
13. Sopantyadvayamantyam (सोपान्त्यद्वमन्त्यम्)
अन्तिम और उपान्तिम का दुगुना।
‘Ultimate and twice the penultimate’
Corollary: Gunitasamuccayah Samuccayagunitah
14. Ekanyunena Purvena (एकन्यूनेन पूर्वेण)
पहले से एक कम के द्वारा।
‘By one less than the previous one’
Corollary: Dhwajanka
15. Gunitasamuchayah (गुणितसमुच्चयः)
गुणितों का समुच्चय।
‘The whole product (The product of the sums)’
Corollary: Dwandwa Yoga
16. Gunakasamuchayah (गुणकसमुच्चयः)
गुणकों का समुच्चय।
‘Set of multipliers (All the multipliers)’
Corollary: Adyam Antyam Madhyam
1. Anurupyena (आनुरूप्येण)
अनुपातों से।
‘Proportionality’
2. Sisyate-Sesasmjnah (शिष्यते शेषसंज्ञः)
एक विशिष्ट अनुपात में भाजक के बढ़ने पर भजनफल उसी अनुपात में कम होता हैं तथा शेषफल अपरिवर्तित रहता हैं।
‘Quotient decreases in same ratio as divisor increases and remainder remain constant’
3. Adyamadyen –Antyamantyena (आद्यमाद्येन अन्त्यमन्त्येन)
प्रथम को प्रथम के द्वारा तथा अन्तिम को अन्तिम के द्वारा।
‘The first by the first and the last by the last’
4. Kevalaih saptakam-Gunyat (केवलैः सप्तकं गुण्यात्)
7 के लिए गुणक 143
‘For 7 the Multiplicand is 143’
5. Vestanam (वेष्टनम्)
आश्लेषण करके।
‘By ousculation’
6. Yavadunam Tavadunam (यावदूनम् तावदूनम्)
विचलन घटा करके।
‘Subtract by the deficiency’
7. Yavadunam Tavadunikrtya Varganca Yojayet (यावदूनम् तावदूनीकृत्य वर्ग च योजयेत्)
संख्या की आधार से जितनी भी न्यूनता हो उतनी न्यूनता और करके उसी न्यूनता का वर्ग भी रखें।
‘What ever the deficiency subtract that deficit from the number and write along side the square of that deficit’
8. Antyayor Dasake’pi (अन्त्ययोर्दशकेऽपि)
अन्तिम अंकों का योग 10 वाली संख्याओं के लिए।
‘Numbers of which the last digits added up give 10’
9. Antyayoreva (अन्त्ययोरेव)
अन्तिम पद से ही।
‘Only the last terms’
10. Samuchayagunitah (समुच्चयगुणितः)
गुणनफल की गुणन संख्याओं का योग।
‘The sum of the products’
11. LopanaSthapanabhyam (लोपनस्थापनाभयाम्)
विलोपन तथा स्थापना से।
‘By alternate elimination and retention’
12. Vilokanam (विलोकनम्)
देखकर।
‘By mere observation’
13. Gunita Samuccayah Samuccaya Gunitah (गुणितसमुच्चयः समुच्चयगुणितः)
गुणनखण्ड़ो की गुणन संख्याओं के योग का गुणनफल गुणनफल की गुणन संख्याओं के योग के समान होता हैं।
‘The product of the sum of the coefficients in the factors is equal to the sum of the coefficients in the product (The product of the sum is the sum of the products)’
14. Dhwajank (ध्वजांक्):
ध्वज लगाकर।
‘On the flag’
Ekadhikena Purvena (एकाधिकेन पूर्वेण)
The Sutra means: “By one more than the previous one”.
पहले से एक अधिक के द्वारा।
1. This sutra is useful to the ‘squaring of numbers ending in 5’.
Example: 252.
For the number 25, the last digit is 5 and the 'previous' digit is 2. According to formula 'One more than the previous one', that is, 2+1=3. The Sutra gives the procedure 'to multiply the previous digit 2 (by one more than itself) by 3. It becomes the L.H.S (left hand side) of the result, that is, 2 X 3 = 6. The R.H.S (right hand side) of the result is 52, that is, 25.
252 = 2 X 3 / 25 = 6/25=625.
152 = 1 X (1+1) /25 =225;
952 = 9 X 10/25 = 9025;
1352 = 13 X 14/25 = 18225;
2. Vulgar fractions whose denominators are numbers ending in Nine
We take examples of 1 / a9, where a = 1, 2………… In the conversion of vulgar fractions into recurring decimals, Ekadhika process can be effectively used both in division and multiplication.
Division Method: Value of 1 / 19.
The numbers of decimal places before repetition is the difference of numerator and denominator, 19 -1=18 places. For the denominator 19, the purva (previous) is 1. Hence Ekadhikena purva (one more than the previous) is 1 + 1 = 2.
1. 0.10 (Divide numerator 1 by 20, 0 times, 1 remainder)
2. 0.005 (Divide 10 by 2, 5 times, No remainder)
3. 0.0512 (Divide 5 by 2, 2 times, 1 remainder)
4. 0.0526 (Divide 12 or12 by 2, 6 times, No remainder)
5. 0.05263 (Divide 6 by 2, 3 times, No remainder)
6. 0.0526311(Divide 3 by 2, 1 time, 1 remainder)
7. 0.05263115 (Divide 11 or 11 by 25 times, 1 remainder)
8. 0.052631517 (Divide 15 or 15 by 2, 7 times, 1 remainder)
9. 0.0526315718 (Divide 17 or 17 by 2, 8 times, 1 remainder)
10. 0.0526315789 (Divide 18 or 18 by 2, 9 times, No remainder)
11. 0.052631578914 (Divide 9 by 2, 4 times, 1 remainder)
12. 0.052631578947 (Divide 14 or 14 by 2, 7 times, No remainder)
13. 0.05263157894713 (Divide 7 by 2, 3 times, 1 remainder)
14. 0.052631578947316 (Divide 13 or 13 by 2, 6 times, 1 remainder)
15. 0.052631578947368 (Divide 16 or 16 by 2, 8 times, No remainder)
16. 0.0526315789473684 (Divide 8 by 2, 4 times, No remainder)
17. 0.05263157894736842 (Divide 4 by 2, 2 times, No remainder)
18. 0.052631578947368421 (Divide 2 by 2, 1 time, No remainder)
0
Multiplication Method: Value of 1 / 19
For 1 / 19, 'previous' of 19 is 1. And one more than of it, is 1 + 1 = 2. Therefore 2 is the multiplier for the conversion. We write the last digit in the numerator (अंश) as 1 and follow the steps leftwards.
1. 1
2. 21(multiply 1 by 2, put to left)
3. 421(multiply 2 by 2, put to left)
4. 8421(multiply 4 by 2, put to left)
5. 168421 (multiply 8 by 2=16, 1 carried over, 6 put to left)
6. 1368421 (6 X 2 =12, +1 = 13, 1 carried over, 3 put to left)
7. 7368421 (3 X 2, = 6 +1 = 7, put to left)
8. 147368421 (7 X 2 =14, 1 carried over, 4 put to left)
9. 947368421 (4 X 2, = 8, +1 = 9, put to left)
10. 18947368421(9 X 2 =18, 1 carried over, 8 put to left)
11. 178947368421(8 X 2 =16,+1=17, 1 carried over, 7 put to left)
12. 1578947368421(7 X 2 =14,+1=15, 1 carried over, 5 put to left)
13. 11578947368421(5 X 2 =10,+1=11,1 carried over, 1 put to left)
14. 31578947368421(1 X 2 =2,+1=3, 3 put to left)
15. 631578947368421(3 X 2 =6, 6 put to left)
16. 12631578947368421(6 X 2 =12, 1 carried over, 2 put to left)
17. 52631578947368421(2 X 2 =4,+1=5, 5 put to left)
18. 1052631578947368421(5 X 2 =10, 1 carried over, 0 put to left)
Now from step 18 onwards the same numbers and order towards left continue.
Thus 1 / 19 = 0.052631578947368421
Example: Value of 1 / 7.
1/7 में हर के अंक को 9 बनाने के लिए हर और अंश में 7 से गुणा करते हैं।
(1/7 = 7/49); हर के 49 का पूर्वेण हैं, 4; जिसका एकाधिक 5 हैं। भिन्न के आवृति दशमलव स्वरूप का अन्तिम अंक 7 होगा तथा 7-1 = 6 अंकों के पश्चात् दशमलव अंकों की पुनरावृति होगी।
1. 7
2. 357 (multiply 7 by 5 =35, 3 carried over, 5 put to left)
3. 2857 (5 X 5 =25, +3 = 28, 2 carried over, 8 put to left)
4. 42857 (8 X 5 =40, +2 = 42, 4 carried over, 2 put to left)
5. 142857 (2 X 5 =10, +4 = 14, 1 carried over, 4 put to left)
6. 2142857 (4 X 5 =20, +1 = 21, 2 carried over, 1 put to left)
अतः
Nikhilam Navatas’charamam Dasatah (निखिलम् नवतश्चरमं दशतः)
The formula simply means: “all from 9 and the last from 10”
सभी नौ में से परन्तु अन्तिम दस में से।
Example: 1000 - 368 = 632
We subtract each figure of 368 from 9 and the last figure from 10.
हम संख्या 368 के सभी अंकों को 9 में से तथा अन्तिम अंक 8 को 10 में से घटाते हैं।

The formula can be very effectively applied in multiplication of numbers, which are nearer to bases 10, 100, 1000 or to the powers of 10 (for example: 96 x 98 or 102 x 104).
जब दो संख्याएँ आधार 10 , 100 या 10 की धात के निकट हो तो उनका गुणनफल सूत्र निखिलम् द्वारा ज्ञात किया जाता हैं।
i. When both the numbers are lower than the base.
Find 92 X 86. Here base is 100. Now follow the rules, 92 is 8 less than base 100. And 86 is 14 less than the same base 100. Hence 8 and 14 are called deviations from the base.

ii. When both the numbers are higher than the base.
Here the deviation is positive as the numbers are higher then base.

We consider 03x07=21. This is done because, we need to consider two digits in deviation as it the base 100 has two zeros. If the deviation is near 1000 then we need to consider 3 digits in the deviation (e.g., 004 and not just 4).
iii. One number is more and the other is less than the base.
In this situation one deviation is positive and the other is negative. So the product of deviations becomes negative. So the right hand side of the answer obtained will therefore have to be subtracted.

12/ (-8) =112 how?
12/ (-8) should be read as 'one two, eight bar'. Here 'one' and ' two ' are in normal form. ‘Eight' is in complement form (परम मित्र रुप में). So, when we bring a carry from normal form to complement form, '12' becomes '11' and 'eight bar' becomes '2'( complement of 8). Hence 12/ (-8) =112.
Anurupyena (आनुरूप्येण)
The upa-Sutra 'Anurupyena' means 'proportionality' or 'similarly'.
अनुपातों से।
इस सूत्र के उपयोग से आनुपातिक गुणन या भाग किया जाता हैं। जब संख्याऐं सैद्धान्तिक आधार 100 से काफी दूर हो तो क्रियात्मक आधार उपयोग में लाया जाता हैं।
This Sutra is highly useful to find products of two numbers when both of them are near the Common bases like 50, 100 etc (multiples of powers of 10).
Example: 47 X 42
As per the previous methods, if we select 100 as base we get
47 -53
42 -58
This is much more difficult. Now by ‘Anurupyena’ we take a different working base through we can solve the problem. Take the nearest higher multiple of 10. In this case it is 50.
Treat it as 100 / 2 = 50.
1. We choose the working base. Working base is 100 / 2 = 50
2. Write the numbers one below the other
47
42
__
3. Write the differences from 50 against each number on right side
47 -03
42 -08
________
4. Write cross-subtraction or cross- addition as the case may be under the line drawn. Multiply the differences and write the product in the left side of the answer.

5. Since base is 100 / 2 = 50, 39 in the answer represent 39X50.
Hence divide 39 by 2 (because 50 = 100 / 2). Thus 39 ÷ 2 gives 19½ where 19 is quotient and ½ is remainder. This ½, as Reminder gives 50; making the L.H.S of the answer, 24 + 50 = 74 or (½ x 100 + 28) i.e. R.H.S. 19 and L.H.S. 74 together give the answer 1974.
Urdhva Tiryagbhyam (ऊर्ध्व तिर्यग्भ्याम्)
It means “Vertically and cross wise.”
सीधे और तिरछे दोनो प्रकार से।
Urdhva – tiryagbhyam is the general formula applicable to all cases of multiplication and also in the division of a large number by another large number.
Example: 12 X 13
The symbols are operated from right to left.
1. Multiply vertically 2X3

2. Multiply crosswise 1X2 and 1X3

3. Multiply vertically 1X1

= 156
The multiplication of 3 digit number with 3 digit number:

Adyamadyena-Antyamantyena (आद्यमाद्येन अन्त्यमन्त्येन)
The Sutra “Adyamadyena-Antyamantyena” means “the first by the first and the last by the last”.
प्रथम को प्रथम के द्वारा तथा अन्तिम को अन्तिम के द्वारा।
Area of rectangle:
Example:
Find out the area of a rectangle whose length and breadth are respectively 5 ft.2 inches and 4 ft.5 inches.
Generally we continue the problem like this.
Area = Length X Breadth
= 5’ 2" X 4’ 5" (Since 1’ = 12")
= (5 X 12 + 2) (4 X 12 + 5) conversion in to single unit
= 62" X 53" = 3286 Sq. inches.
Since 1 sq. ft. =12 X 12 = 144 sq.inches
We have area
3286 /144 = Quotient is 22 and Remainder is 118.
Area of rectangle is 22 Sq. ft 118 Sq. inches.
Mental argumentation:
It is interesting to know the mental argumentation. It goes in his mind like this
5’ 2"
4’ 5"
First by first: 5’ X 4’ = 20 sq. ft.
Last by last: 2" X 5" = 10 sq. in.
Now cross wise 5 X 5 + 4 x 2 = 25 +8 = 33.
Adjust units to left as 33 = 2 X 12 +9 , 2 twelve's as 2 square feet make the first 20+2 = 22 sq. ft ; 9 left becomes 9 x 12 square inches and go towards right 9 x 12 = 108 sq. in. gives 108+10= 118 sq.inch.
We got area in some sort of 22 sq ft and 128 sq. inches.
By Vedic principles "the first by first and the last by last"
5’ 2" can be treated as 5a + 2 and 4’ 5" as 4a + 5,
Where a= 1ft. = 12 inch and a2 = 1 sq. ft = 144 sq. inch.
= (5a + 2) (4a + 5)
= 20a2 + 25a + 8a + 10
= 20a2 + 33a + 10
= 20a2 + (24a+9a) + 10
= 20a2+ (2a+9) a + 10 writing 33 = 2X12 +9
= 22a2+ 9a + 10
= 22 sq. ft. + 9X12 sq. inch + 10 sq. inches
= 22 sq. ft. + 108 sq. inch + 10 sq. inches
= 22 sq. ft. + 118 sq. inch
Factorization of quadratics:
By Vedic process two sub-sutras are used to factorizing a quadratic.
(a) Anurupyena (b) Adyamadyena-Antyamantyena
The usual procedure of factorizing a quadratic is as follows:
= 2 a2 + 9a + 10
= 2 a2 + 4a + 5a + 10
= 2a (a + 2) + 5 (a + 2)
= (a + 2) (2a + 5)
But by mental process, we can get the result immediately. The steps are as follows.
1. Split the middle coefficient in to two such parts that the ratio of the first coefficient to the first part is the same as the ratio of the second part to the last coefficient. Thus we split the coefficient of middle term of 2 a2 + 9a + 10 i.e. 9 in to two such parts 4 and 5 such that the ratio of the first coefficient to the first part of the middle coefficient i.e. 2:4 and the ratio of the second pat to the last coefficient, i.e. 5: 10 are the same. It is clear that 2:4 = 5:10. Hence such split is valid. Now the ratio 2: 4 = 5: 10 = 1:2 give one factor (a+2).
2. Second factor is obtained by dividing the first coefficient of the quadratic by the first coefficient of the factor already found and the last coefficient of the quadratic by the last coefficient of the factor. i.e. the second factor is
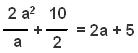
2 a2 + 9a + 10 = (a + 2) (2a + 5)
Sankalana – Vyavakalanabhyam (संकलन-व्यवकलनाभ्याम्)
This Sutra means: by addition and by subtraction.
जोड़ने और घटाने के द्वारा।
This sutra is widely used in solving a simultaneous equation where the coefficients of algebraic value are found interchanged.
Example:
84a + 41b = 166 (1)
41a + 84b = 209 (2)
With the help of Sankalana – vyavakalanabhyam
Add equation (1) and (2)
125a + 125b = 375
125 (a + b) = 375
a + b =3 (3)
Subtract equation (1) from (2)
43a - 43b = -43
43 (a – b) = -43
a – b = -1 (4)
Adding equation (3) and (4)
2a = 2
a = 1
Subtracting equation (3) from (4)
2b = 4
b = 4 /2
b = 2
Hence a = 1 and b = 2
Example:
7a + 3b = 13 (1)
3a + 7b = 17 (2)
समीकरण (1) व (2) को जोड़ने पर
10a + 10b = 30
a + b =3 (3)
समीकरण (1) व (2) को घटाने पर
4a - 4b = -4
a – b = -1 (4)
समीकरण (3) व (4) को जोड़ने पर
2a = 2
a =1 (3)
समीकरण (3) व (4) को घटाने पर
2b = 4
b = 2
अतः a = 1 and b = 2
Yavdunam Taavdunikritya Vargancha Yojayet (यावदूनम् तावदूनीकृत्य बर्ग च योजयेत)
This sutra means “What ever the deficiency subtract that deficit from the number and write along side the square of that deficit.”
संख्या की आधार से जितनी न्यूनता हो उसमे उतनी न्यूनता और करके उसी न्यूनता का वर्ग भी रखे।
This sutra is used to calculate squares of numbers near (lesser) to powers of 10
Example: 982
1. The nearest power of 10 to 98 is 100.
2. We take 100 as our base.
3. Since 98 is 2 less than 100, hence deficiency is 2.
4. We decrease the number by an amount equal to the deficiency which is (98 -2) = 96. This is the left side of our answer.
5. On the right hand side put the square of the deficiency. That is square of 2 = 04.
6. Hence the answer is 9604.
While calculating step 5, the number of digits in the squared number (04) should be equal to number of zeroes in the base (100). Hence in our case, the base 100 has 2 zeros and hence square of 2 is 04 and not just 4.
Example: 962.
1. 96, 100 के पास हैं।
2. अतः आधार 100 लेते हैं।
3. आधार से न्यूनता = 4
4. 96 में से 4 घटाते हैं। (96 - 4 ) = 92 जो उत्तर का बायाँ भाग होगा।
5. 4 का वर्ग करते हैं, 42 = 16 ( 2 digits) जो उत्तर का दायाँ भाग होगा।
6. पद 4 तथा 5 के परीणाम को साथ में रखने पर 962 = 9216 प्राप्त होता हैं।
Yavadadhikam Taavadhikikritya Vargancha Yojayet (यावद्धिकम् तावद्धिकृत्य वर्ग च योजयेत)
This sutra means “whatever the extent of its surplus, increment it to that very extent; write along side the square of that extent.”
संख्या की आधार से जितनी अधिकता हो उसमें उतनी अधिकता और करके उसी अधिकता का वर्ग भी रखें।
This sutra is very useful in calculating the squares of numbers nearer (greater) to powers of 10.
इस सूत्र का उपयोग उन संख्याओं का वर्ग ज्ञात करने में किया जाता हैं, जो 10 की घात से थोड़ी बड़ी हो।
Example: 1092
1. 109, 100 के पास हैं।
2. हम आधार 100 लेते हैं।
3. आधार से अधिकता = 9
4. 109 में 9 जोड़ते हैं, (109+9 ) = 118 जो उत्तर का बायाँ भाग होगा।
5. अब 9 का वर्ग करते हैं, 92 = 81, जो उत्तर का दायाँ भाग होगा।
6. उत्तर के दायें भाग में उतने ही अंक रखते हैं, जितने आधार में शून्य हो। यदि अंक कम या अधिक हो तो उन्हें समायोजित करते हैं।
7. पद 4 तथा 5 के परीणाम को साथ में रखने पर 1092 = 11881 प्राप्त होता हैं।
Example: 1042
1. The nearest power of 10 to 104 is 100.
2. We take 100 as our base.
3. Since 104 is 4 more than 100, hence surplus is 2.
4. We increase the number by an amount equal to the surplus which is (104 +4) = 108. This is the left side of our answer.
5. On the right hand side put the square of the surplus. That is square of 4 = 16.
6. Hence the answer is 10816.
Antyayor Dasakepi (अन्त्ययोर्दशकेऽपि)
The Sutra means - numbers of which the last digits added up give 10.
अन्तिम अंकों का योग 10 वाली संख्याओं के लिए।
जिन अंकों के चरम (अन्तिम) अंकों का योग 10 या 10 की घात हो तथा शेष निखिलम् अंक समान हो, उनकी गुणन संक्रिया इस विधि द्वारा की जाती हैं।
15 and 15, 1 is common and 5 + 5 = 10
57 and 53, 5 is common and 7 + 3 = 10
82 and 88, 8 is common and 2 + 8 = 10
126 and 124, 12 is common and 6 + 4 = 10
425 and 475, 4 is common and 25 + 75 = 100
Example: 32 X 38
32 X 38
= 3 x 4 / 2 x 8
= 12 /16
= 1216
1. Sum of last digits is 2+8 =10,
2. Remaining digits =3 are same in both numbers.
3. RHS 2x8 =16,
4. LHS 3 x (3+1) =12
Example: 83 X 87
83 X 87
= 8 x 9 / 3 x 7
= 72 /21
= 7221
1. चरमं अंकों का योग = 3+7 =10,
2. शेष निखिलम् अंक समान =8
3. दायाँ पक्ष = 3 x 7 =21,
4. बायाँ पक्ष = 8 x (8+1) =72
Lopana Sthapanabhyam (लोपनस्थापनाभ्याम्)
The sutra means 'by alternate elimination and retention’.
विलोपन तथा स्थापना से।
This sutra is used to factorizing a quadratic equation of type ax2+by2+cz2+dxy+eyz+fzx. It is a homogeneous equation of second degree with three variables x, y, z.
इस सूत्र का उपयोग द्विघात समीकरण के गुणनखण्ड़ करने में किया जाता हैं।
Example: 3a2 + 6b2+ c2 +11ab + 5bc + 4ac
1. सर्वप्रथम c=0; रखकर c को विलोपित करते हैं, तथा आद्यमाद्येन अन्त्यमन्त्येन सूत्र की सहायता से गुणनखण्ड़ करते हैं।
3a2 +11ab + 6b2
= 3a2 +9ab +2ab + 6b2
= (3a + 2b) (a + 3b)
2. अब b=0; रखकर b को विलोपित करते हैं, तथा आद्यमाद्येन अन्त्यमन्त्येन सूत्र की सहायता से गुणनखण्ड़ करते हैं।
3a2 + 4ac + c2
= 3a2 +3ac + ac + c2
= (3a + c) (a + c)
3. इन दो गुणनखण्ड़ समूह की सहायता से विलोपन के कारण आई रिक्तियों की पूर्ति करते हैं।
= (3a + 2b +c) (a + 3b +c)
Example: 2a2 + 6b2+ c2 +7ab + 5bc + 3ac
1. Eliminate c and retain a, b; factorize
2a2 + 7ab + 6b2 = (2a + 3b) (a + 2b)
2. Eliminate b and retain a, c; factorize
2a2 + 3ac + c2 = (2a + c) (a + c)
3. Fill the gaps, the given expression
= (2a + 3b + c) (a + 2b + c)
Gunita Samuccayah - Samuccaya Gunitah (गुणितसमुच्चयः समुच्चयगुणितः)
The product of the sum of the coefficients in the factors is equal to the sum of the coefficients in the product (i.e., the product of the sum is the sum of the products).
गुणनखण्ड़ों की गुणन संख्याओं के योग का गुणनफल, गुणनफल की गुणन संख्याओं के योग के समान होता हैं।
This sutra is useful for the factorization of quadratic expressions.
Example: (2a + 1) (3a + 5) = 6a2 + 13a + 5
Here: (2 + 1) (3 + 5) = (6 + 13 + 5)
= 24: Thus verified.
Example: (x + 5) (x + 7) (x - 2) = x3 + 10x2 + 11x – 70
(1 + 5) (1 + 7) (1 - 2) = 1 + 10 + 11 – 70
6 x 8 x -1 = 22 – 70
– 48 = – 48 Verified
Paravartya-yojayet (परावर्त्य योजयेत्)
‘Paravartya – Yojayet’ means 'transpose and apply'
चिन्ह परिवर्तित कीजिये तथा संक्रिया प्रारम्भ कीजिये।
Simple division of algebra
Example: Divide (a3 – 3a2 + 10a – 4) by (a – 5)

1. (a3 / a) gives a2, 1 the first coefficient in the Quotient.
2. Multiply 1 by + 5, (obtained after reversing the sign of second term in the Quotient) and add to the next coefficient in the dividend. It gives 1 X (+5) = +5, adding to the next coefficient, i.e., –3 + 5 = 2, this is second coefficient in Quotient.
3. Multiply 2 by +5, i.e., 2 X +5 =10, add to the next coefficient 10 + 10 = 20. This is third coefficient in Quotient.
4. Thus Quotient is a2 + 2a + 20
5. Now multiply 20 by + 5 =100. Add to the next (last) term, 100 + (-4) = 96, which becomes R, i.e., R =96.
Example: Divide (2a5 + a3 – 3a + 7) by (a3 + 2a – 3)
We treat the dividend as (2a5 + 0a4 + 1a3+ 0a2 – 3a + 7) and divisor as (a3 + 0a2 + 2a - 3).

क्रियाविधिः
1. भाजक के प्रथम पद को छोड़कर शेष पदों के गुणकों के चिन्ह बदलकर परावर्तित अंक प्राप्त करते हैं, जो क्रमशः 0, -2, +3 हैं।
2. 2a5 में a3 का भाग देने पर 2a2 आता हैं, अतः भागफल का प्रथम गुणक =2;
3. भागफल का प्रथम गुणक अंक (2) X परावर्तित अंक (0) = 0, मध्य खण्ड़ के +0a4 के नीचे 0 लिखते हैं, (2) X (-2) = -4, मध्य खण्ड़ के +1a3 के नीचे -4 लिखते हैं, तथा (2) X (+3) = +6, तृतीय खण्ड़ के +0a2 के नीचे +6 लिखते हैं।
4. भाजक के द्वितीय पद का योग = 0 + 0 = 0, अतः भागफल का दूसरा गुणक = 0;
5. भागफल का दूसरा गुणक अंक (0) X परावर्तित अंक (0) = 0, मध्य खण्ड़ के +1a3 के नीचे 0 लिखते हैं, (0) X (-2) = 0, तृतीय खण्ड़ के 0a2 के नीचे 0 लिखते हैं, तथा (0) X (+3) = 0, तृतीय खणड़ के -3a के नीचे 0 लिखते हैं।
6. योग = 1 - 4 + 0 = -3, अतः भागफल का तीसरा गुणक = -3;
7. भागफल का तीसरा गुणक अंक (-3) X परावर्तित अंक (0) = 0, तृतीय खण्ड़ के 0a2 के नीचे 0 लिखते हैं, (-3) X (-2) = +6, तृतीय खण्ड़ के -3a के नीचे +6 लिखते हैं, तथा (-3) X (-3) = +9, तृतीय खणड़ के +7 के नीचे +9 लिखते हैं।
8. अतः भागफल = 2a2 - 3; शेषफल = + 6a2 + 3a -2
Paravartya in solving simple equations:
'Paravartya yojayet' means 'transpose and apply'. According to the rule invariable change its sign with every change of side from left to right, (+) becomes (-) and; and (X) becomes (÷). Further it can be extended from numerator to denominator in the concerned problems.
प्रत्येक पक्षान्तरण में गणितीय राशियों के चिन्ह परिवर्तित होते हैं। इस प्रकार (+) चिन्ह (-) हो जाता हैं व (-) चिन्ह (+) हो जाता हैं, (x) का (÷) व (÷) का (x) हो जाता हैं।
Application 1: If ax + b = cx + d.
By paravartya, we get-

Example: 4x + 3 = 2x + 9
Here a =4, b = 3, c = 2, d = 9

Application 2: If (x + a) (x +b) = (x +c) (x +d).
By paravartya, we get -

Example: (x + 7) (x + 9) = (x - 8) (x - 11).
Here a =7, b = 9, c = - 8, d = -11

Application 3: If

By paravartya, we get-

Example:

Application (4): If
By paravartya we get-
Example:

Application (5): If

Example:

Application (6): If

Example:

Simple equations:
By Paravartya sutra we can derive the values of x and y. which are given in two simple equations.
Example:
2x + 3y = 13,
4x + 5y = 23.
1. x का मान ज्ञात करने के लिए दोनों समीकरण के y के गुणक तथा अचर राशियों का बज्र गुणा करते हैं, तथा बज्र गुणा से प्राप्त राशियों को घटाते हैं, प्राप्त संख्या x के लिए अंश के रुप में प्रयुक्त होती हैं।
2x + 3y = 13
4x + 5y = 23
“X” के लिए अंश
= 3 x 23 – 5 x 13
= 69 – 65 = 4
2. दोनों समीकरणों के x तथा y के गुणक का बज्र गुणा कर घटाने पर प्राप्त संख्या x के लिए हर के रुप में प्रयुक्त होती हैं।
“X” के लिए हर
= (3 x 4) – (2 x 5)
= 12 – 10 = 2
अतः X = 4 ÷ 2 = 2
3. y का मान ज्ञात करने के लिए दोनों समीकरण के x के गुणक तथा अचर राशियों का बज्र गुणा करते हैं, तथा बज्र गुणा से प्राप्त राशियों को घटाते हैं, प्राप्त संख्या y के लिए अंश के रुप में प्रयुक्त होती हैं।
“Y” के लिए अंश
= (13 x 4) – (23 x 2)
= 52 – 46 = 6
4. y के लिए हर = 2; जो पद 2 से प्राप्त हुआ।
अतः y = 6÷2 = 3
अतः समीकरण में, x = 2 तथा y = 3
Sunyam Samyasamuccaye (शून्यं साम्यसमुच्चये)
The Sutra 'Sunyam Samyasamuccaye' means 'Samuccaya is the same, that Samuccaya is Zero.' The term 'Samuccaya' has several meanings under different contexts.
'जब समुच्चय एक समान हो तो उस समुच्चय का मान शून्य होता हैं'। भिन्न-भिन्न परिस्थितियों में समुच्चय के अर्थ भिन्न-भिन्न होते हैं।
Situation 1: “Samuccaya” as a term which occurs as a common factor in all the terms concerned and proceed as follows.
यदि समीकरण के प्रत्येक पद में x एक सर्वनिष्ट खण्ड़ हो तो x = 0 होगा।
Example: The equation 12x + 3x = 4x + 5x has the same factor “x” in all its terms. Hence according to the sutra it is zero.
12x + 3x = 4x + 5x
x = 0
Example: In 2(x+1) = 7(x+1)
(x + 1) is Common Samuccaya
Hence (x + 1) = 0
x = -1
Situation 2: If the product of independent terms in a equations like (x+a) (x+b) = (x+c) (x+d), is same then x = 0;
यदि समीकरण के दोनों पक्षों में अचर राशियों रहित (स्वतंत्र) पद समान हो तो x का मान शून्य (0) होगा।
Example: (x + 3) (2x + 5) = -3(x - 5)
Samuccaya is 3 x 5 = 15 = -3 x -5
Since it is same, Hence x = 0
Situation 3: “Samuccaya” as the sum of the denominators of two fractions having the same numerical numerator.
यदि समीकरण में दो भिन्नों के अंश परस्पर समान हो तो उनके हरों का योग शून्य रखने पर चर राशि का मान प्राप्त होता हैं।
Example:

Numerator are same = j;
Hence according to sutra, sum of the denominators is zero.
(2x + 1 + 3x + 4) = 0
= (5x + 5) = 0
5x = -5
x = -1
Situation 4: If the sum of the numerators and the sum of the denominators are same, then that sum = 0.
यदि समीकरण में दोनों पक्षों के अंशों तथा हरों का योग परस्पर समान या एक निश्चित अनुपात में हो तो उनके अंशों तथा हरों का योग शून्य रखने पर चर राशि का मान प्राप्त होता हैं।
Example:

Sum of numerator = 2x+3+2x+5 = 4x + 8
Sum of denominator =2x+5+2x+3 = 4x + 8
Hence according to sutra, sum is zero
(4x + 8) = 0
4x = -8
x = -2
Example:

दोनों पक्षों के अंशों का योग = 3x+4+x+1 = 4x+5
दोनों पक्षों के हरों का योग = 6x+7+2x+3 = 8x+10
दोनों पक्षों का अनुपात = 1 : 2
सूत्र के अनुसार
(4x +5) = 0
4x = -5
x = -5/4
Situation 5: If the differences of the numerators and denominators of each side are same, then that difference = 0.
यदि समीकरण में एक पक्ष के अंश तथा हर का अन्तर दूसरे पक्ष के अंश तथा हर के अन्तर के समान या एक निश्चित अनुपात में हो तो किसी भी अन्तर का मान शून्य रखने पर चर राशि का मान प्राप्त होता हैं।
Example:

बायें पक्ष के अंश व हर का अन्तर = (3x+6) – (6x+3)
= - 3x + 3 = - (3x-3)
दायें पक्ष के अंश व हर का अन्तर = (5x+4) – (2x+7)
= 3x - 3
सूत्र के अनुसार
3x - 3 = 0
3x = 3
x = 1
Situation 6: ‘Samuccaya’ with the same sense but in a different context and application.
यदि समीकरण के प्रत्येक पक्ष में दो पद हो और पद का प्रत्येक अंश परस्पर समान हो तथा दोनों पक्षों के हरों का योग समान हो तो योग को शून्य रखने पर चर राशि का मान प्राप्त होता हैं।
Example:

बायें पक्ष के हरों का योग = x+2+x+6 = 2x+8
दायें पक्ष के हरों का योग = x+1+x+7 = 2x+8
सूत्र के अनूसार
(2x + 8) = 0
2x = -8
x = -4
Sunyam Samyasamuccaye in Cubes:
Example: (x – 6)3 + (x – 8)3 = 2 (x – 7)3
Traditional method,
(x – 6)3 + (x – 8)3 = 2 (x – 7)3
x3 – 18x2 + 108x – 216 + x3 – 24x2 + 192x – 512
= 2 (x3 – 21x2 + 147x – 343)
2x3 – 42x2 + 300x – 728 = 2x3 – 42x2 + 294x – 686
300x – 728 = 294x – 686
300x – 194x = 728 – 686
6x = 42
x = 42 / 6 = 7
Vedic method,
We have (x – 6) + (x – 8) = 2x – 14. Taking out the numerical factor 2, we have (x – 7) = 0
According to “Sunyam Samyasamuccaye” (x – 7) = 0.
Hence x = 7
Anurupye– Shunyamanyat (आनुरूप्ये शून्यमन्यत्)
The Sutra means: 'If one is in ratio, the other one is zero'.
अनुरूपता होने पर दूसरा शून्य होता हैं।
This Sutra in used to solve simultaneous simple equations in which the coefficients of 'one' variable are in the same ratio to each other as the independent terms are to each other. In such a case the Sutra says the 'other' variable is zero.
यदि किसी युगपत् समीकरण के किसी एक चर का अनुपात अचर राशियों के अनुपात के समान हो तो दूसरा चर शून्य होगा।
Example:
3x + 4y = 1
4x + 12y = 3
The ratio of y-coefficients is 4:12 = 1:3, which is same as the ratio of independent terms is = 1:3.
Hence the other variable x = 0
4y = 1 or 12y = 3
y = ¼
Example:
175x + 140y = 350
350x + 324y = 700
X के गुणकों का अनुपात = 175:350 =1:2 तथा
अचर पदों का अनुपात =350:700 = 1:2 समान हैं,
अतः y = 0
(175x =350) or (350x =700)
x = 2
Puranapuranabhyam (पूरणापूरणाभ्याम्)
The Sutra can be taken as Puranapuranabhyam which means by the completion or non - completion. We use it to solve the roots of quadratic equation.
अपूर्ण को पूर्ण करके।
इस सूत्र का प्रयोग द्विघात तथा त्रिघात समीकरणों को हल करने में किया जाता हैं।
ax2 + bx + c = 0
x2 + (b/a)x + c/a = 0 ( dividing by a )
x2 + (b/a)x = - c/a
Completing the square (purana) on L.H.S
[x2 + (b/a)x + (b2/4a2)] = -c/a + (b2/4a2)
[x + (b/2a)] 2 = (b2 - 4ac) / 4a2

Example: x3 + 6x2 + 11 x + 6 = 0.
क्योंकि (x + 2) 3 = x3 + 6x2 + 12x + 8
दोनों पक्षों में (x + 2) जोड़ते हैं।
x3 + 6x2 + 11x + 6 + (x + 2) = x + 2
x3 + 6x2 + 12x + 8 = x + 2
(x + 2) 3 = (x + 2)
a3 = a for (a = x + 2)
a = 0, a = 1, a = - 1
x + 2 = 0, 1,-1
x = -2,-1,-3
Chalana - Kalanabhyam (चलन-कलनाभ्याम्)
The Sutra means 'Sequential motion' or “By calculus”.
चलन कलन के द्वारा।
Application 1: It is used to find the roots of a quadratic equation
(x2 – 3x + 1) = 0.
Now by calculus formula:

2x–3 = ±√5
x = 3±√5 / 2
Every Quadratic can thus be broken down into two binomial factors.
Application 2: Gunak samuccaye: यदि द्विघात समीकरण ax2 + bx + c, किन्ही दो पदों का गुणनफल हैं, तो इसकी प्रथम अवकलन गुणन संख्या दोनों गुणनखण्ड़ों का योग होती हैं।
ax2 + bx +c
= (x + d) (x +e)
By calculus formula:

Sutra says 2ax + b = (x + d) + (x +e)
Example:
x2 + 5x + 4 =(x+4) (x+1)
(2x + 5) =(x+4) + (x+1)
Ekanyunena Purvena (एकन्यूनेन पूर्वेण)
This Sutra is a Sub-sutra to Nikhilam which means 'by one less than the previous one’.
पहले से एक कम के द्वारा।
This sutra is used to multiply a number by 9, 99, 999...
दो गुणन संख्याओं में जब एक संख्या के सभी अंक 9 हो तो एकन्यूनेन पूर्वेण विधि द्वारा गुणा किया जाता हैं। जिस संख्या के सभी अंक 9 हो उसे गुणक तथा दूसरी संख्या को गुण्य कहते हैं।
Example: 11 x 99
11 X 99
= 11-1 / 99-10
= 10 / 89
= 1089
1. बायाँ पक्ष= 11 - 1 = 10
2. दायाँ पक्ष = 99-10 = 89
Example: 125 X 9
1. Divide the multiplicand (125) of by a Vertical line or by the Sign (:) put as many digits as the multiplier into a right hand portion.
125 has to be written as 12/5 or 12:5
2. Subtract from the multiplicand one more than the whole excess portion on the left.
Left portion of multiplicand is 12. One more than it 12 + 1 = 13,
Now subtract this from multiplicand,
3. Subtract the R.H.S. part of the multiplicand by nikhilam process.
R.H.S of multiplicand is 5 its nikhilam is 5.
It gives the R.H.S of the product
Answer is 11: 2: 5 = 1125

Antyayoreva (अन्त्ययोरेव)
'Antyayoreva' means 'only the last terms'.
अन्तिम पद से ही।
This is useful in solving simple equations. The type of equations are those whose numerator and denominator on the L.H.S. leaving the independent terms stand in the same ratio to each other as the entire numerator and the entire denominator of the R.H.S. stand to each other.
यह सूत्र उन समीकरणों को सरल करने में प्रयुक्त होता हैं, जिनके बायें पक्ष में अन्तिम पदों ( स्वतंत्र पदों) को छोड़कर अंश तथा हर का अनुपात वही होता है, जो दायें पक्ष के पूरे अंश तथा हर का होता हैं। इसे अन्त्ययोरेव अर्थात् अन्तिम पदों के अनुपात द्वारा आसानी से हल किया जा सकता हैं।
Example:
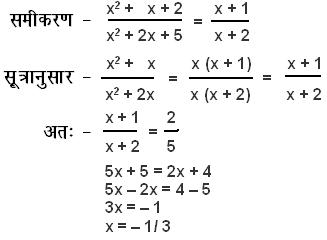
Example: (a + 2) (a + 3) (a + 11) = (a + 4) (a + 5) (a + 7)
Sum of the binomials on both the L.H.S. and R.H.S = 3a + 16 are same. Hence antyayoreva can be applied. Adjusting we get

Vilokanam (विलोकनम्)
The Sutra 'Vilokanam' means 'Observation'.
देखकर।
Example: x + (1/x) = 10/3
उक्त समीकरण में विलोकनम् के अनुसार बायाँ पक्ष दो व्युत्क्रमों (x तथा 1/x) का योग हैं। साथ ही दायाँ पक्ष भी दो व्युत्क्रमों (3 तथा 1/3) का योग हैं।
अतः x = 3, 1/3.
Example:

Simultaneous Quadratic Equations:
Example: 5x – y = 7 and xy = 6.
xy = 6 gives
x = 6, y = 1;
x = 1, y = 6;
x = 2, y = 3;
x = 3, y = 2 and of course negatives of all these.
Observe that
For x = 6, y = 1; 5x – y = 5 (6) – 1 = 30 – 1 ≠ 7.
For x = 1, y = 6; 5x – y = 5 (1) – 6 = 5 – 6 ≠ 7.
For x = 3, y = 2; 5x – y = 5 (3) – 2 = 15 – 2 ≠ 7.
These set are not solutions because they do not satisfy the equation 5x – y = 7.
But for x = 2, y = 3; 5x – y = 5 (2) – 3 = 10 – 3 = 7
Hence x = 2, y = 3 is a solution.
Negative values of the above are also not the solutions. Thus one set of the solutions is x = 2, y = 3.
Partial Fractions:
Example:
















































No comments:
Post a Comment